TECHNIQUES FOR FOLDING PAPER INTO A PRIME NUMBER OF EQUAL SECTIONS
1)Twos are the easiest sections to fold, you simply fold the paper over until no paper is left uncovered ,in other words fold the paper in half or end to end.

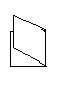
2)To fold threes fold the paper over until the paper left uncovered equals approximately the paper covered, then fold the uncovered paper back over the covered paper.
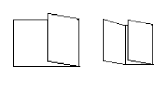
3)To fold five's fold the paper over until the paper left uncovered equals approximately half the covered paper, then fold the covered paper in half, and last fold the uncovered paper back over the covering and covered paper.
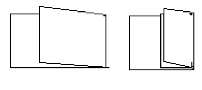
4)To fold sevens fold the paper until the paper left uncovered equals approximately one third of the covered paper, then fold the covering and covered paper into threes as shown above in technique (3), and last fold the uncovered paper back over the covered paper.
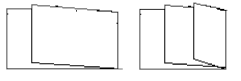
TECHNIQUES FOR FOLDING PAPER INTO A COMPOSITE NUMBER
OF EQUAL SECTIONSTo fold paper into a number of sections equal to two raised to some power (n) simply fold the paper in half (n) times. For example eight equals 2 raised by a power of 3 , so you will fold the paper in half three times

Folding even numbers of sections, other than powers of two, will include some folds equal 2 raised by a power of (n) with combinations of other prime folds shown above. For example twelve equals 4 times 3 equals (2 raised by a power of 2) times 3, so fold the paper in half 2 times, then into threes as shown above.

Folding a odd composite number of sections requires combinations of the prime number folds. An example is fifteen or 3 times 5. In general it is best to start with the bigger number, so fold the paper into five sections, and then into three sections.
These folding strategies are equivalent to the prime factoring of composite numbers. The magnitude of numbers you can factor is increased by folding the paper in one direction and then in the other direction to multiply two numbers. For example 48 equals 8 times 6, first fold eight in one direction of the paper, by folding the paper in half 3 times (2*2*2), then fold six across the eight in the other direction, by folding the paper in half and then into thirds(2*3). Therefore the prime factors of 48 are (2*2*2)*(2*3). I found the hands on folding process made prime factoring easier for children having difficulty.

In some cases I have children write the number of sections for a technique on the first fold line of the technique, in this way keeping track of the prime numbers as they fold.